Dynamics of quadratic family: from attracting fixed point to absolutely continuous invariant probability (ACIP)

February 21, 2010

Dynamics of quadratic family: from attracting fixed point to absolutely continuous invariant probability (ACIP)

Filed under: Dynamical Systems — Zhenghe @ 9:30 pm
Tags: acip, Henon map, Jacobson theorem, periodic doubling, quadratic family

This is one of my previous notes, I post it for testing:)

In study of Smooth Ergodic Theory, we always follow the way that geometrical properties of derivative implies statistical properties of dynamical systems.

Typical statistical behavior of dynamical systems is hyperbolicity, which includes uniform hyperbolicity, partial hyperbolicity and nonuniform hyperbolicity. Famous examples of uniformly hyperbolic systems are linear automorphisms on 2-torus and geodesic flows, while partially hyperbolic systems are given by time-1 map of Anosov flows and Frame flows. Both uniform hyperbolicity and partial hyperbolicity are open conditions.

Then how about nonuniform hyperbolicity? First of all, it is not an open condition. And there is few good example. Furthermore, we can ask ‘are typical systems nonuniformly hyperbolic?’. Here I am going to introduce an famous example related to nonuniformly hyperbolic theory, which gave rise to nice generalization.

First, let us introduce the so-called Henon map, which is given by $f_{a,b}(x,y)=(x^2-a+y, bx)$ . It was given by Henon in 1976 and he found some strange attractor when a=1.4, b=0.3. Then followed by Benedicks, Carleson 1991 and Benedicks, Young 1993’s work, we have the following theorem:

Theorem: Let $f_{a,b}(x,y)=(x^{2}-a+y,bx)$ , then $\forall b\neq 0$ sufficiently small, there exist $\Omega^{*}(b)$ satisfies

(a) $\forall a\in\Omega^*(b)$ , $f_{a,b}$ is nonuniformly hyperbolic.
(b) $m(\Omega^*(b))>0$ , and there is no interval in $\Omega^{*}(b)$

Concretely, here nonuniform hyperbolicity means for every pair of a, b above, there exists an attractor $\Lambda_{a,b}$ with ergodic SRB measure with nonzero Lypapunov exponents and $\Lambda_{a,b}$ contains tagencies.

Henon map is the two dimensional case, its study was originated from the one dimensional case, namely, b=0 and $f_a(x)=x^2-a$ the quadratic family, where we have the following theorem, which is known as Jacobson theorem

Theorem(Jacobson, 1981):
There exists $\Omega^*$ , s.t. i) $\forall a\in\Omega^*$ , $f_a$ is nonuniformly hyperbolic
(which means it has ergodic SRB measure which is absolutely continuous w.r.t. Lebesgue measure, and positive Lyapunov exponent); ii) $m(\Omega^*)>0$ and contains no intervals.

Now we are going to focus our study on the quadratic family $f_a(x)=x^2-a$ . First, we will study the change of dynamical behavior of $f_a$ when parameter $a$ varies.

Let $f_{a}=x^{2}-a$ , $\varepsilon>0$ is a sufficiently small number, $p_{1}(a)\leq p_{0}(a)$ are two roots of equation $x^{2}-a=x$ and $I_{a}=[-p_{0}(a), p_{0}(a)]$ , then then we have the following description:

Let $a_{0}$ be the number that $x^{2}-a_{0}=x$ has a unique solution, then

If $a<a_{0}$ , then $\forall x\in R$ , $f_{a}^{n}(x)\rightarrow\infty$ when $n\rightarrow\infty$

If $a=a_{0}$ then:
$f_{a}^{n}(x)\rightarrow p_{1}(a), \forall x\in I_{a}$
$f_{a}^{n}(x)\rightarrow\infty, \forall x\notin I_{a}$

If $a=a_{0}+\varepsilon$ then:
$f_{a}^{n}(x)\rightarrow p_{1}(a), \forall x\in I_{a}$
$f_{a}^{n}(x)\rightarrow\infty, \forall x\notin I_{a}$

Let $a_{1}$ be the number that $f'_{a_{1}}(p_{1}(a_{1}))=-1$ then:

If $a=a_{1}-\varepsilon$ then:
$f_{a}^{n}(x)\rightarrow p_{1}(a), \forall x\in I_{a}$
$f_{a}^{n}(x)\rightarrow\infty, \forall x\notin I_{a}$

If $a=a_{1}+\varepsilon$ , then $f'_{a_{1}}(p_{1}(a))<-1$ so there is no fix point. But the graph of $y=f^{2}_{a}(x)$ and y=x will intersect in four points and one of them is an attracting fix point. So $f_{a}$ will have an attracting 2-periodic orbit such that $\forall x\in I, f^{n}_{a}(x)$ will approach this orbit.

Then following the idea of renormalization, we can take part of the graph of $f^{2}_{a}$ and analyze it just as those of $f_{a}$ for $a>a_{1}$ . Then we will find that the attracting 2-periodic orbit will preserve until a reaches an $a_{2}$ . For $a>a_{2}$ , we consider $f^{4}_{a}$ , then we will find a fix point which is an attracting 4-periodic orbit of $f_{a}$ .

Keep going this way, we will find a sequence $\{a_{i}\}_{i\geq0}$ such that for every $a_{i}$ , there will be a doubling of period of attracting orbit. In the end, $\{a_{i}\}_{i\geq0}$ will converge to a number $a_{\infty}<2$ .

$a=a_{\infty}$ is the so-called infinitely renormalizable case, it was dicovered by Feigenbanm in 1970s. In this case, $f_{a}$ has an invariant interval $I$ , for almost all $x\in I, f^{n}_{a}(x)\rightarrow C$ , where C is an invariant Cantor set and the dynamical behavior on C just likes the irrational rotation of unit circle.

For $a=2$ ,Ulam-Von Neumann found the behavior of $f_{a}(x)=x^{2}-a$ on $[-2, 2]$ is conjugate with the tent map T on $[0,1]$ via map $h(z)=2\cos(\pi z)$ , where T is given by

$T(x)=-2x+1$ for $0\leq x\leq \frac{1}{2}$
$T(x)=2x-1$ for $\frac{1}{2}< x\leq1.$

That is, $f_{2}=h\circ T\circ h^{-1}$ . But we know that the Lebesgue measure $m$ of $[0, 1]$ is the ergodic and invariant measure of tent map $T$ . So the smooth map $h$ will push $m$ forward to be a ergodic acip (absolutely continuous invariant probability) $\mu$ of $f_{2}$ on $[-2, 2]$ . But for one dimensional map with single critical point, existence of acip implies positive Lyapunov exponent. So $f_{2}$ is a nonuniformly hyperbolic system on $[-2, 2]$ and $\mu$ is its SRB measure (in one dimensional case SRB coinsides with acip).

When $a>2$ , there will be no invariant interval of $f_{a}$ , but there does exist an invariant Cantor set on $I_{a}$ such that the dynamical behavior on it is topologically conjugate with the left shift map on $\Sigma_{2}=\{0, 1\}^{N}$ .

Now we are going to study the parameter interval $(a_{\infty}, 2)$ , which has rich phenomena and deep results. For example, there are lots of parameters with attracting periodic orbits which give rise to many cascades of doubling bifurcation which just like the process from $a_0$ to $a_{\infty}$ . Actually, Simo and Tatjer numerically found about 30 million “periodic windows” with period $\leq 24$ , and the total length of them is about $10\%$ of $(a_{\infty}, 2)$ .

If we let $\Omega= (a_{\infty}, 2)$ , $\Omega^-= \{a: f_a$ admits attracting periodic orbits $\}$ and $\Omega^+=\{a: f_a$ admits acip $\}$ , then we have the following serial deep results:

Theorem(Jacobson, 1981):
$m(\Omega^+)>0$ .

Theorem(Luzzatto, Takahashi, 2006):
$m(\Omega^+)>10^{-5000}$ .

Theorem(Craczjk, Smotek, Lyubich, 1997):
$\Omega^-$ is open and dense in $\Omega$ .

Theorem(Lyubich, 2002):
$\Omega^+\bigcup\Omega^-$ has full measure of $\Omega$ .

Remark:
1) $(a_{\infty}, 2)\setminus (\Omega^{+}\bigcup\Omega^{-})$ is not empty, for example, there are infinitely renormalizable systems and systems whose physical measure support on repelling fixed point.

2) Generalization: first, $m(\Omega^+)>0$ for the following one dimensional maps: Multimodel map(Tsujii, 1993), Contracting Lorenz map(Rovella, 1993), Lorenz like map with critical point(Luzzatto and Tacker 2000) and infinite critical points map(Pacifer, Parclla and Viena, 2000); second, $\Omega^-$ is open and dense in space of $C^r$ maps $(r\geq1)$ (Kozlosky, Shen and Von Stier, 2005).

Comments (2)

2 Comments »

A question: how about the entropy of f_a for a\in(a_\infty,2)?

Comment by Pengfei — February 23, 2010 @ 5:16 am | Reply
- Good question: If a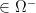 $\in\Omega^-$ then obviously entropy is zero and if a $\in\Omega^+$ then since it admits a SRB measure,
  the entropy is nothing than the integral of Lyapunov exponent w.r.t. the SRB measure which is an ACIP
  
  Comment by zhenghe — February 23, 2010 @ 5:46 am | Reply

RSS feed for comments on this post. TrackBack URI

Zhenghe's Blog

February 21, 2010